Cymatics is the process of visualizing sound by transferring vibrations to a medium such as water, sand, or a thin plate. When sound frequencies are played, they cause the medium to vibrate, forming patterns that correspond to the specific sound frequency. The resulting patterns are typically symmetrical and become more complex with higher frequencies. Cymatics allows for the observation of the physical patterns produced by sound waves.
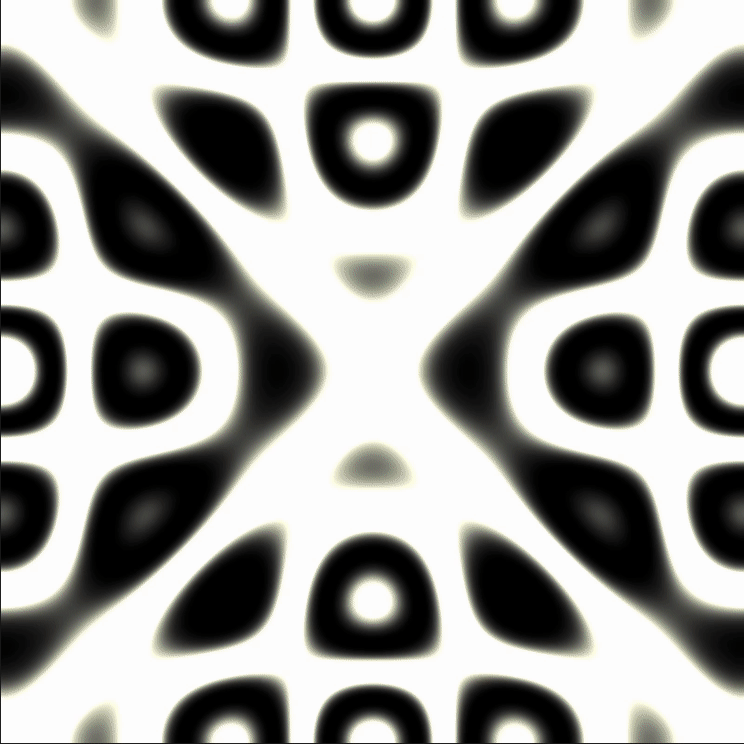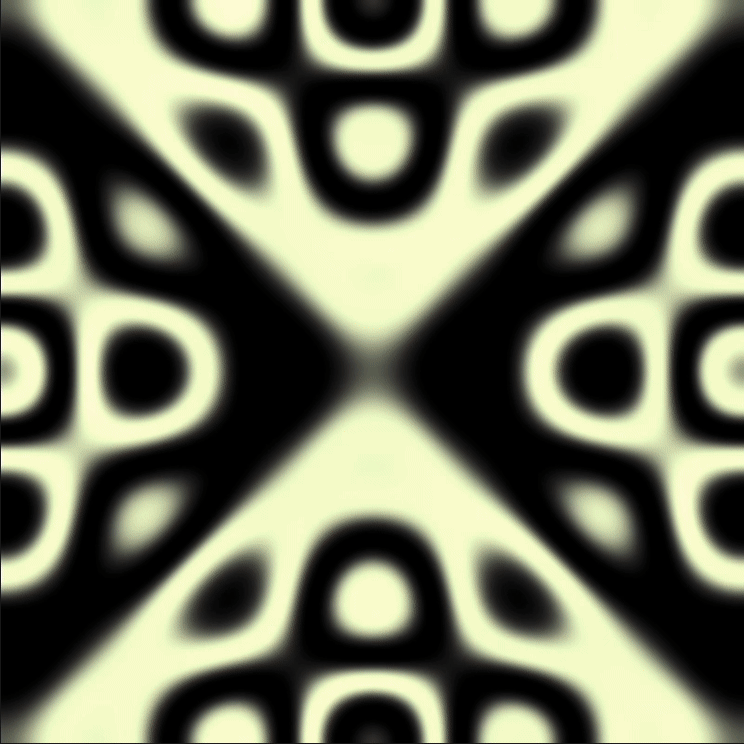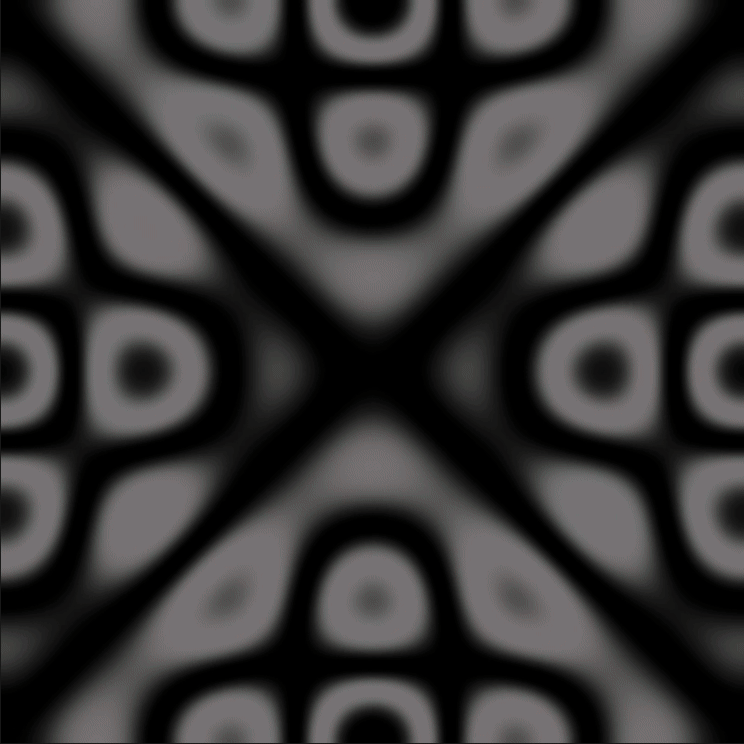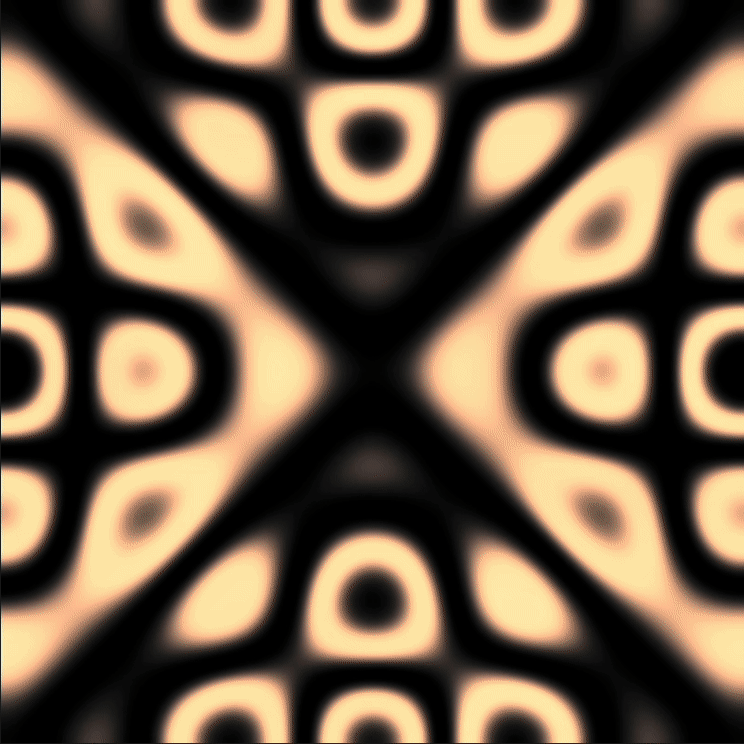

Examples of conventional cymatic parameters
In mathematics, a field is a function that is evaluated at a point in space. In the realm of physics, a field is a region in which each point is affected by a force. Fields can be gravitational, electromagnetic, or other types.
Here, field functions are applied to the input parameters (n, m, L) of the cymatic equation.
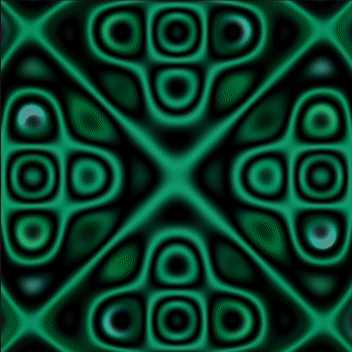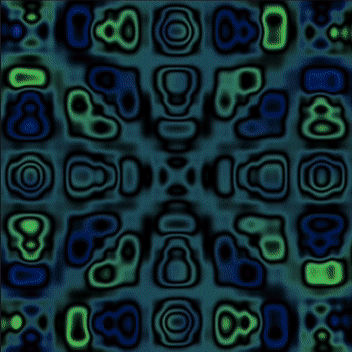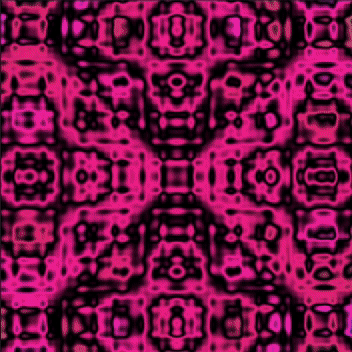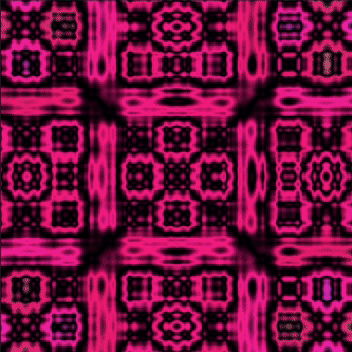

Field functions based on quantum wave interference



Field functions composed of layered Perlin-like noise
According to contemporary Quantum Field Theory (QFT), fields are not isolated entities. They overlap and interpenetrate each other in space. This means that at any given point, multiple fields can coexist and influence each other.
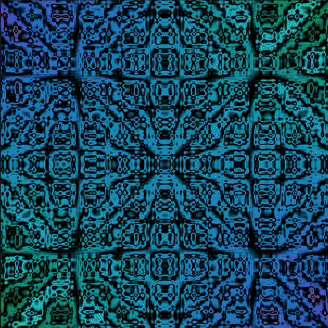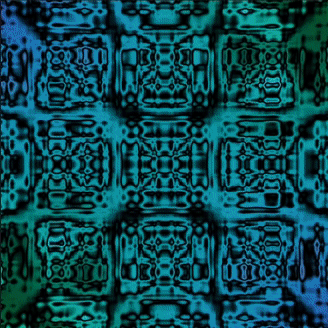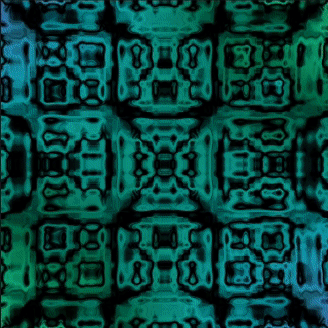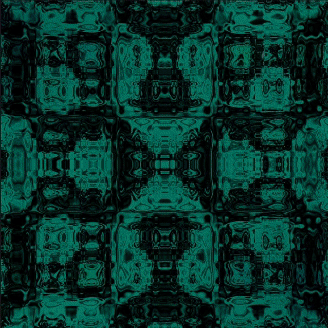
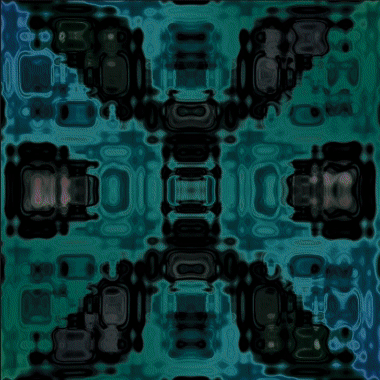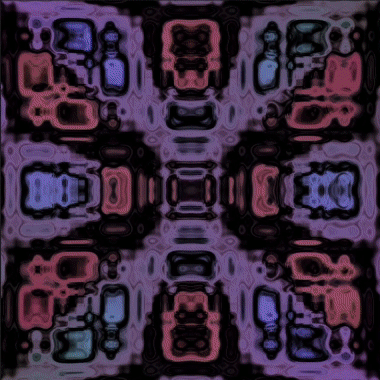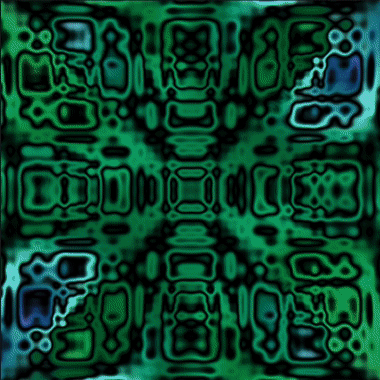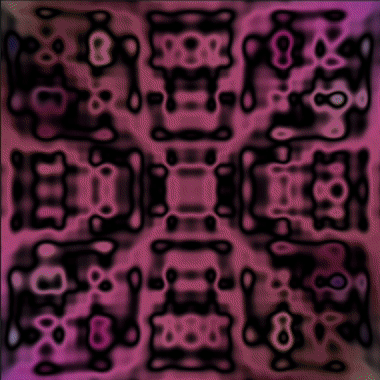

The output of the cymatic equation interpreted through multiple nested fields
When fields are applied to the cymatic at multiple levels (i.e., to the input parameters and to the resultant output), new intricate patterns emerge.
Demonstration of the Cymatic Fields sketch
Experiments with style transfer